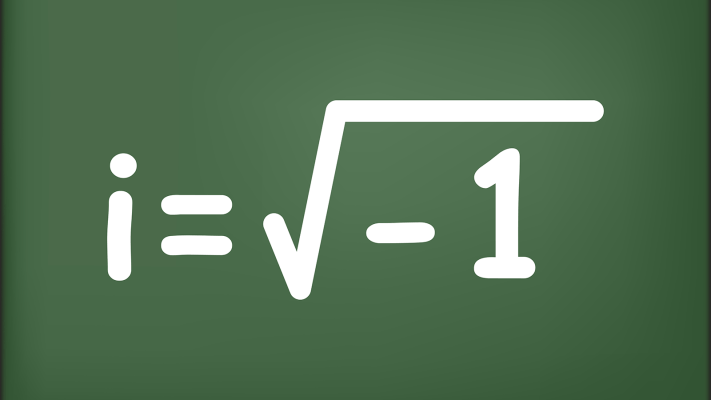
Imaginary numbers are a mathematical concept that extends the set of real numbers. They are numbers that involve the imaginary unit, denoted as “i,” which is defined as the square root of -1. In other words, imaginary numbers are multiples of the imaginary unit.
Imaginary numbers are not “real” in the sense of representing physical quantities, but they have significant applications in various branches of mathematics and science, particularly in complex analysis, electrical engineering, and quantum mechanics.
Here are some key points about imaginary numbers:
- Definition of the Imaginary Unit:
- The imaginary unit, denoted as “i,” is defined as the square root of -1: i^2 = -1.
- This definition allows for the creation of numbers that involve the imaginary unit.
- Imaginary Numbers:
- Imaginary numbers are expressed in the form a + bi, where “a” and “b” are real numbers, and “i” represents the imaginary unit.
- “a” represents the real part of the number, while “b” represents the imaginary part.
- For example, 3i, -5i, and 2 + 4i are examples of imaginary numbers.
- Imaginary Unit Properties:
- Multiplying the imaginary unit by itself gives -1: i * i = -1.
- Dividing any real number by the imaginary unit gives a pure imaginary number: a / i = -ai.
- Complex Numbers:
- Complex numbers are numbers that combine both real and imaginary parts.
- They are expressed in the form a + bi, where “a” is the real part and “b” is the imaginary part.
- Complex numbers encompass both real numbers (where b = 0) and imaginary numbers (where a = 0).
- Complex Plane:
- Complex numbers can be represented geometrically on a complex plane, which consists of a horizontal axis (representing the real part) and a vertical axis (representing the imaginary part).
- The position of a complex number on the complex plane corresponds to its real and imaginary parts.
Imaginary numbers provide a powerful mathematical tool for solving various equations and problems that involve complex quantities. They are an important concept in mathematics and find applications in many scientific and engineering fields.
History of imaginary numbers:
The concept of imaginary numbers has a rich and intriguing history that spans several centuries. Here’s a brief overview of the development and understanding of imaginary numbers:
- Origins:
- The roots of imaginary numbers can be traced back to ancient civilizations such as the Babylonians and Egyptians, who worked with mathematical problems involving square roots of negative numbers. However, these early civilizations did not develop a formal system for handling such numbers.
- Renaissance Mathematics:
- The notion of imaginary numbers began to take shape during the Renaissance period in Europe.
- Italian mathematician Gerolamo Cardano (1501-1576) encountered square roots of negative numbers while solving cubic equations but considered them as “fictitious” or “imaginary” solutions.
- Rafael Bombelli:
- The true understanding of imaginary numbers took a significant step forward with the work of Italian mathematician Rafael Bombelli (1526-1572).
- Bombelli recognized that negative square roots could be manipulated algebraically, even though they did not have a direct interpretation in terms of real-world quantities.
- He introduced the concept of “imaginary numbers” and developed rules for their operations, treating them as valid mathematical entities.
- Complex Numbers:
- The foundation of the modern understanding of imaginary numbers was laid by the Swiss mathematician Leonhard Euler (1707-1783) and the Irish mathematician William Rowan Hamilton (1805-1865).
- Euler introduced the notation “i” to represent the imaginary unit, and he established the famous Euler’s formula linking exponential functions, imaginary numbers, and trigonometric functions: e^(ix) = cos(x) + i * sin(x).
- Hamilton further developed the concept of complex numbers, which combine both real and imaginary parts, and introduced the term “imaginary” for the square root of negative numbers.
- Acceptance and Further Development:
- Initially, the idea of imaginary numbers faced skepticism and resistance from mathematicians.
- However, as mathematicians recognized their importance and applicability, the understanding and acceptance of imaginary numbers gradually grew.
- The development of complex analysis in the 19th century, led by mathematicians like Augustin-Louis Cauchy and Bernhard Riemann, solidified the theoretical foundation of imaginary and complex numbers.
Today, imaginary numbers are considered an integral part of mathematics and are widely used in fields such as engineering, physics, computer science, and more. The historical journey of imaginary numbers highlights the ongoing evolution of mathematical concepts and the human quest for deeper understanding.
